Masoud Kamgarpour: The Job Hunt
I graduated from University of Chicago in 2008. My advisor was Drinfeld. I am currently a faculty member in Australia. I am visiting UChicago to talk about job hunting for mathematics students. I did my studies/postdocs in four different countries: Canada, USA, Germany, and Australia. I am familiar with the advantages and disadvantages of each. I had a lot of ups and downs in my career, but ended up with a job that I love. In addition, I am quite familiar with the attitudes of UChicago students regarding job market, etc. I am hoping that sharing my experience will help fellow UChicago graduate students plan their future better.
Annual AWM Postdoc Panel
Postdocs Agnes Beaudry, Hung Vinh Tran, Ronen Mukamel, and Davide A. Reduzzi will share their wisdom about the job search process, adjusting to being a postdoc, and life after the postdoc. This is a great opportunity for both younger grad students and those nearing graduation to learn about the academic career path, and to get their questions answered.
Valia Gazaki: Impossibility theorems for indefinite integrals
We all know that the real function $e^{-x^2}$ does not have “an elementary antiderivative”. Other non elementary integrals are the famous elliptic integrals. But what does it mean to be non elementary? In this talk, we will define what it means to be integrated in elementary terms and give two criteria for a function not to have an elementary antiderivative. We will use these to prove that $\int e^{-x^2}\,dx$ is not an elemantary integral. All we will need is a little bit of field theory and complex analysis.
Raluca Havarneanu: The Grothendieck–Riemann–Roch Theorem
I will explain Grothendieck’s approach to proving the theorem for a proper morphism between two quasi-projective varieties over a field.
Clark Butler: Shadows
I will explain the phenomenon of orbit shadowing in hyperbolic dynamics with an example.
Ben Fehrman: Bring The Noise
We’ll discuss how to describe systems which admit no description. At least not that I’d screw with. Not in this lifetime. Give up and frown upon Brownian motion. Derive Ito’s formula, aka the fundamental theorem of stochastic calculus, aka flex mcnasty, aka the 37th chamber, and affix a precise intuition to its mystical reality. Finally, describe the connection between differential equations and stochastic processes, better understand the processes and recall the beginning.
Max Engelstein: Can you hear the shape of a … windchime? Xylophone?
The interplay between functional analysis and geometry is a rich subject which has produced some of the crown jewels of 20th century mathematics. But I don’t like the word “bundle”, so we will do spectral graph theory instead. There will be pictures of graphs, analysis without deltas or epsilons and some hand-waving towards big fancy theorems at the end.
Sergei Sagatov: Classical Mechanics and Symplectic Geometry
We explain via the canonical formalism of Hamilton why symplectic geometry is the natural mathematical framework for classical mechanics and prove a classical result of Noether relating symmetries and conserved quantities.
Tori Akin: Dictatorships disguised as democracies
A cute application of ultrafilters.
Bena Tshishiku: Shocking Card Trick Revealed!
Mathematician stuns audience with magic trick. What happens next will surprise you!
Henry Scher: Supersymmetry — a graphical representation
Ever wanted to learn what supersymmetry is really all about? Well, this isn’t really going to explain it, but it’s going to give you just enough that you can pretend to! We’ll start with a pseudo-explanation of quantum mechanics, turn to supersymmetry, and end up with the concept of adinkras.
All material beyond quantum mechanics comes from papers by Sylvester James Gates.
Jonathan Wang: Number theory in real life — algebraic curves and codes
Error correcting codes are used when transmitting messages through noisy channels. For example, the ISBN is a code consisting of nine digits $a_i$ followed by a digit 0–9 or X corresponding to $\sum_i a_i \pmod{11}$. I will start by giving the definition of a code and proceed to give a few examples, including the binary Hamming code, which will be constructed using polynomials over a finite field. The Hamming code turns out to be an example of a Goppa code. These latter codes are constructed using linear systems on curves over finite fields. It turns out that curves with many rational points relative to their genus give rise to good codes. The Riemann Hypothesis gives a preliminary bound on the number of rational points for curves of fixed genus. Other bounds are discussed, and we will see how class field theory helps us come up with “good” codes.
Short version: see some cool arithmetic geometry applied to [a layman’s] real life. Get some ideas for what to write in your next NSF grant.
Henry Chan: When finite spaces met homotopy theory
Have you seen a finite topological space? If so, do you know that they can have infinitely many non-trivial homotopy groups? Buckle up, because these spaces are gonna be legend … wait for it …
Tianqi Fan: Zariski’s main theorem
This is an introduction to Zariski’s main theorem.
Daniel Studenmund: Theorem? I Hardly Know ’Em!
We’ve all heard of the Four Color Theorem. Blah blah longstanding open problem blah blah computer-assisted proof blah. But did you know that it is equivalent to the fact that every snark is non-planar? (If so, you’d better bring some reading material.) This talk will cover some basic coloring problems in graph theory with a topological slant.
Paul Apisa: The Icosahedral Solution to the Quintic
It’s one of those truly galling things to hear: Klein solved the quintic with an icosahedron in 1884. It makes it sound like Klein was playing D&D; in the basement of Gottingen when suddenly he glanced at the die and chuckled “My God, of course that’s how it’s done!”
We’ll start by talking about classifying $3$-manifolds by their fundamental groups, content ourselves with the finite fundamental group case, realize that we’ve stumbled into an ADE classification, then use some uber-classical algebraic geometry (Segre embedding, doubly ruled quadrics) to reduce solving the quintic to things we’ve serendipitously just discussed + the computation of some polynomial invariants. As per math talk etiquette, I will not compute in public.
Sean Howe: Hyperbol(e)ic Number Theory
The number $x^2 + x + 41$ is prime for all $x$! Hyperbolic manifolds have no rational points! A million proofs that there are no imaginary quadratic fields of class number $1$! Unravel these mysteries, and more, this week at *drumroll* *cartwheels* *lion taming* PIZZA SEMINAR!
Katie Mann: The Schwarzian Derivative
The Schwarzian is a terrible looking formula but actually a beautiful piece of math. Come find out why I (geometer, general-calculus-hater-at-large) think it’s a delightful idea to spend a whole hour talking about something that involves third derivatives.
This talk starts with words like “what are the isometries of the circle?” and ends with words like “cocycle on the Virasoro lie algebra of smooth vector fields” and “complex structure on Teichmuller space”. In the middle, we’ll see some classical projective geometry (turns out that shit is really cool!) and a few other surprises.
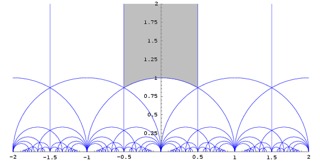
Preston Wake: Sums of squares
Given positive integers $n$ and $k$, in how many ways can you write $n$ as the sum of $k$ squares? Looks like a simple enough question, but looks can be deceiving. We’ll discuss some cases of this question and we’ll see some of these pizza-seminar favourites come up: generating functions, class field theory, $L$-functions, quaternions, Fourier transforms, trace formulas, congruence subgroups, modular forms, Riemann–Roch, that picture of the upper-half plane tiled by semi-circle-triangles that everyone always draws, and more!
Jenny Wilson: Generating functions
Despite their elementary foundations, generating functions are amazingly useful tools — with uses like solving recurrence relations, studying sequences’ asymptotics, computing statistics, and proving combinatorial identities. In this talk we’ll see some applications to binomial relations, symmetric group combinatorics, and random walks. There will be an overview of the basic theory for those who want a refresher, and some challenge problems for the more advanced.
Weiyan Chen: Model Theory and Algebra
Often times after a theorem about the complex numbers is proven in a book, a seemingly innocent claim follows: “the result holds equally well for arbitrary algebraically closed field of characteristic zero”. What if the proof uses structures of $\mathbb{C}$ other than being a field (e.g. uses topology or complex analysis)? In this talk, I will talk about the Lefschetz principle which justifies such a statement. This new perspective viewing algebraic objects, quite unexpectedly, comes from logic, or more precisely, model theory.
Subhadip Chowdhury: Cut-Copy-Paste — Algebra and Tiling
There is a long tradition when resolution of geometric questions requires us to
depart the world of geometry and enlist the help of algebra. The oldest go back
to the Greeks who were concerned with trisecting angles and constructing squares
of equal area to a circle! Coming back to the present, we want to consider problems
of the following nature:
“If a chessboard is covered by $21$ blocks of size $1 \times 3$, what are the
possible locations for the remaining square?”
“Is it possible to tile a square with an odd number of triangles,
all of which have the same area?”
As it turns out, the first one ends up being settled with the aid of the group ring and cyclotomic fields, and the second one leads us to call on valuation theory and Sperner’s lemma. In the upcoming talk, we will discuss some of the algebraic methods used to solve such tiling and related problems.
Mary He: Symplectic Lefschetz Fibrations
In this talk, I will explain the connections between Lefschetz fibrations, symplectic $4$-manifolds and mapping class groups. In particular, I will talk about monodromies of symplectic Lefschetz fibrations and factorizations in mapping class groups, and survey some classification results on low-genus Lefschetz fibrations. If time permits, I will present an example due to D. Auroux which suggests a new approach to distinguish homeomorphic surfaces of general type.
Fedor Manin: How to find mistakes in your dictionary
Have you ever wondered how Google Translate works? Well, that’s still a secret. But earlier this year researchers at the Googleplex published several papers on the arxiv in which they mapped words to a high-dimensional vector space and used linear transformations to translate between English-space and, say, Czech-space. Maybe linear algebra won’t supplant the sophisticated statistical models anytime soon, but the authors are shopping it at least as a quick way of scanning dictionaries for questionable entries. Come find out what happens when you project the numbers 1 through 5 onto a plane, and a bit about how neural networks work.
Jacob Perlman: Hat Games and Error Correcting Codes
You and several other players are offered a chance at a game. Each of you will have either a red hat or a black hat placed on your head, uniformly and independently at random, then you will be allowed to see each other but not communicate, finally and simultaneously each person must either guess the color of their hat or pass. If there is at least one correct guess and no incorrect guesses, you each win a million dollars. What’s an optimal strategy, how often does it win, and what does this have to do with Hamming codes? For the purposes of this talk, hats will be replaced with playing cards and \$1,000,000 will be represented by a piece of candy.
Asilata Bapat: Moment maps and Morse theory
A group action on a space (manifold) usually gives us some insight into properties of the space (manifold). The situations get even better if the group, the action, or the manifold is decorated with adjectives such as compact, Lie, algebraic, smooth, symplectic, Hamiltonian, etc. Morse theory is also another powerful tool to extract information about the topology of smooth manifolds. In this talk I will introduce the notion of a moment map for a group action on a symplectic manifold and give a brief survey of what it can be used for. I will also give a quick introduction to Morse(–Bott) theory and talk about connections with the moment map.
Nick Salter and Tim Black: Conway’s Tangle Trick
If we just told you straight up that this week’s pizza seminar talk was going to be
about math and magic tricks, you’d probably think it would be about as cool and/or
interesting as this guy:
 But what if we told you that we’d be talking about a magic trick invented by this guy:
But what if we told you that we’d be talking about a magic trick invented by this guy:
 And that this was some of the math involved:
And that this was some of the math involved:
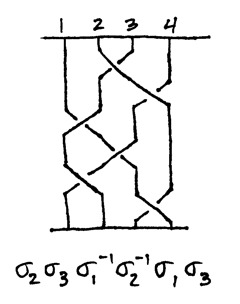
 Then you’d probably want to come.
Then you’d probably want to come.
Ian Frankel: Points, Lines and Planes
We will be talking about low-dimensional affine and projective geometry. In particular, we will interest ourselves in the axiomatic characterizations of projective and affine geometries, how Desargues’s theorem on perspectivity of triangles relates to the classification of projective geometries in higher dimensions, and what goes wrong in dimension $2$.
Daniel Le: Reciprocity Laws, from Diophantus to Langlands
Let $f$ be a polynomial with integer coefficients. How many solutions does $f = 0$ have modulo each prime? The answer is given by reciprocity laws (some established and some conjectural). I’ll begin with several examples before discussing the connection between this question and Galois representations, $L$-functions, and automorphic forms.
